Numerical modeling

The laboratory's research topics on ultrasound require support from various finite element modeling methods, formulated in the frequency domain, in the time domain (spectral methods) for linear or nonlinear propagation, using a modal approach (vibrations, guided waves, periodic media), etc. In this context, a Python library named Elastodynamicsx, dedicated to the simplified use of the versatile Fenicsx library, is being developed by Pierric Mora and made available under the open-source MIT license.

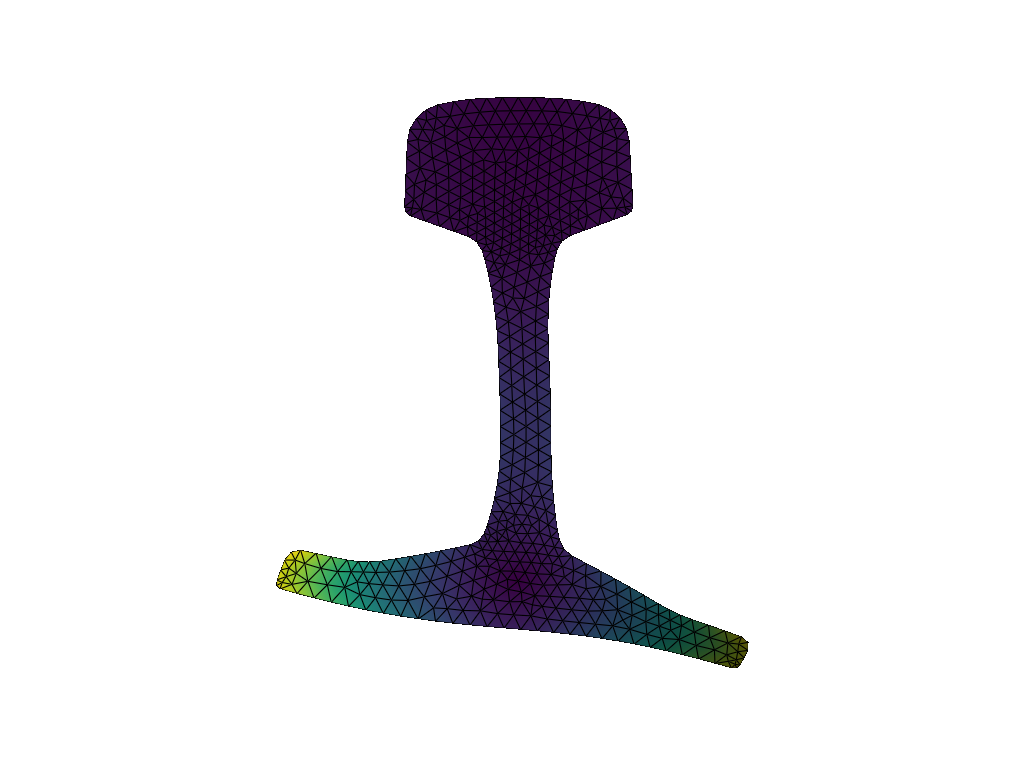
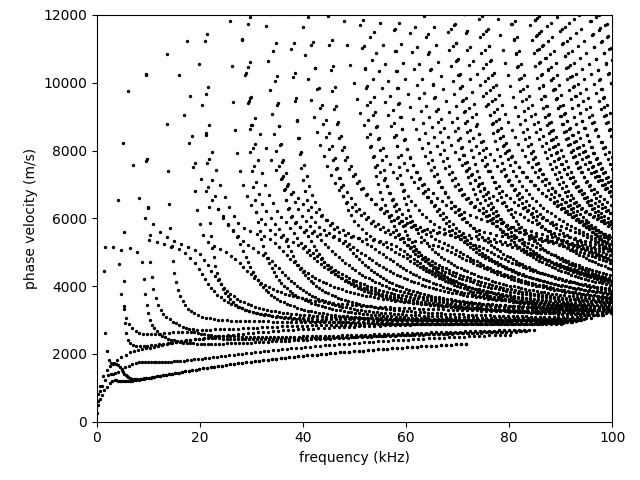
Elongated structures of civil engineering (strands, bars, tubes, rails, anchor rods...) and subsurface (soils, pavements, dikes...) induce a natural wave guidance that allows for long propagation distances with minimal energy losses, meaning the possibility of inspecting large areas with few sensors. However, the complexity of guided wave propagation mechanisms, which are multimodal and dispersive in nature, requires the development of numerical tools dedicated to waveguides, in order to optimize non-destructive testing (NDT) and monitoring techniques.

For that purpose,  a Python library named Waveguicsx for solving complex waveguide problems using the so-called semi-analytical finite element (SAFE method), is developed by F. Treyssède and made freely available under the GNU GPL, version 3, thus providing an accessible solution to the scientific community. Waveguicsx stems from expertise gained over the past 15 years through the guided wave research activity of the laboratory. It can deal with complex waveguides, two-dimensional (e.g. plates) or three-dimensional (arbitrarily shaped cross-section), inhomogeneous in the transverse directions, anisotropic. Complex-valued problems can be handled including the effects of non-propagating modes (evanescent, inhomogeneous), viscoelastic loss (complex material properties) or perfectly matched layers (PML) to simulate buried waveguides.

This waveform inversion method (seismic or radar), tested in several PhD theses (F. Lopes 2009, F. Bretaudeau 2010, R. Valensi 2014), takes into account the entire temporal signal in the tomographic reconstruction. It can lead to a quantitative description of the surveyed medium without necessarily requiring the identification of each wave train (refracted, reflected, direct, guided, etc.). This is particularly useful for processing subsurface waves, where small dimensions result in short temporal separations between wave trains.